Objectives
- Review basic equation for a plane.
- Learn how to find the equation of a line perpendicular to a plane.
- Understand how to find the equation of a line where two planes intersect.
Section 3.4 Planes in Space
In the CalcVR Lesson we explored how to specify a plane using a point and a normal vector to the plane. We review this concept below and then look at activities where we find the equation of a line perpendicular to a plane and discuss the intersection of planes.
Subsection 3.4.1 Planes in Three Dimensions
Given a point \((x_0,y_0,z_0)\) on a plane and a vector \(\mathbf{n} = \langle a,b,c \rangle\) serving as the normal vector for plane, we can form the equation of the plane as follows: For any point \((x,y,z)\) on the plane, the vector with initial point \((x_0,y_0,z_0)\) and terminal point \((x,y,z)\) given by
\begin{equation*}
\mathbf{v} = \langle x - x_0, y-y_0, z-z_0 \rangle
\end{equation*}
must be orthogonal to the normal vector \(\mathbf{n}\text{.}\) This tells us that
\begin{equation*}
0 = \mathbf{n} \cdot \mathbf{v} = \langle a,b,c \rangle \cdot \langle x - x_0, y-y_0, z-z_0 \rangle.
\end{equation*}
Performing the calculation for the dot product above we end up with the basic equation of a plane
\begin{equation}
0 = a(x-x_0) + b(y-y_0) + c(z-z_0).\tag{3.4.1}
\end{equation}
Activity 3.4.1. Perpendicular Line.
Given a plane and a point on the plane, our current goal is to find the equation of a line passing through the point that is perpendicular to the plane.
(a)
Open the CalcVR App and goto the Perpendicular Line activity. In the CalcVR App, you will be shown pictures containing a plane and will answer the following question
- Select the vector that corresponds to the normal vector to the plane. (Answer in CalcVR App)
- Select the line that is perpendicular to the curve. (Answer in CalcVR App)
- At what point do the plane and the line intersect? (Answer in CalcVR App)
- Record the value for the point of intersection and the normal vector in your notebook.
- Comparing the line to the normal vector, what is a direction vector for the line pictured?
(b)
Recall the equation of a line in three dimensions discussed in Subsection 3.3.1. Using the information from the first part of this activity, write down the equation of the line perpendicular to the plane at the given point. Confirm your result using this following tool:
Exercises 3.4.2 Exercises
For the following, find the equation of the line perpendicular to the plane at the specified point. Confirm your results using the tool above.
1.
\(2(x-1)-(y+2)+3(z+3)=0\) at the point \((1,-2,-3)\)
2.
\(4x - 3y + 7z = 7\) at the point \((2,-2,-1)\)
3.
\(4x-5z=2\) at the point \((3,-1,2)\)
Subsection 3.4.3 Intersecting planes
Activity 3.4.2. Intersecting Planes.
Let us now look at what happens when two planes are graphed together.
(a)
Give a geometric description of the three possibilities for the intersection of two planes in three-dimensional space.
(b)
Consider the plane with normal vector \(\mathbf{n} = \langle 2, -1, 3 \rangle\) passing through the point \((-1,2,1)\text{.}\) Write down the equation of the plane using equation (3.4.1).
(c)
The plane from part part b is graphed in yellow below. Consider another plane (that will be graphed in green below) given by
\begin{equation*}
ax+by+cz = 4.
\end{equation*}
How would you pick \(a,b,c\) so that this new plane would not intersect the plane you found in part b? Use the tool below to confirm your result by specifiying the values for \(a, b,\) and \(c\text{.}\) (This will change the graph of the plane colored green.)
(d)
Repeat the previous exercise, choosing different values of \(a,b,c\text{.}\)
(e)
\begin{equation*}
ax+by+cz=d
\end{equation*}
(f)
Based on the previous exercises if two planes completely overlap or do not intersect at all, what can you say about the normal vectors for the planes?
Activity 3.4.3. Intersecting Planes Part 2.
Let us now look at how to find the equation of a line formed by the intersection of planes.
(a)
Consider the following two intersecting planes. What is the geometric relationship between the yellow normal vector for the plane and the red direction vector of the line? Explain why.
(b)
What is the geometric relationship between the green normal vector for the plane and the red direction vector of the line? Explain why.
(c)
Given the geometric relationships above, if you know the normal vectors to your two planes, what is the easiest way to find a direction vector for the line of intersection?
(d)
Consider the following two planes
\begin{equation}
2x-y+3z=4 \qquad \text{and} \qquad x-3y+z=-3.\tag{3.4.2}
\end{equation}
Use what we learned above to find the direction vector for the line of intersection.
(e)
To find the equation of the line all we now need is a single point on the line (see Subsection 3.3.1). Luckily, there are infinitely many to choose from. Observe that for a point to be on the line, the point must be on both planes. Thus the point must satisfy both equations given in equation (3.4.2). Find a point on the line using the equations above. If you are having trouble, you can consult the hint or solution.
Hint.
Pick your favorite \(x\) value between \(-2\) and \(2\) and plug that into the equations for the planes.
Solution.
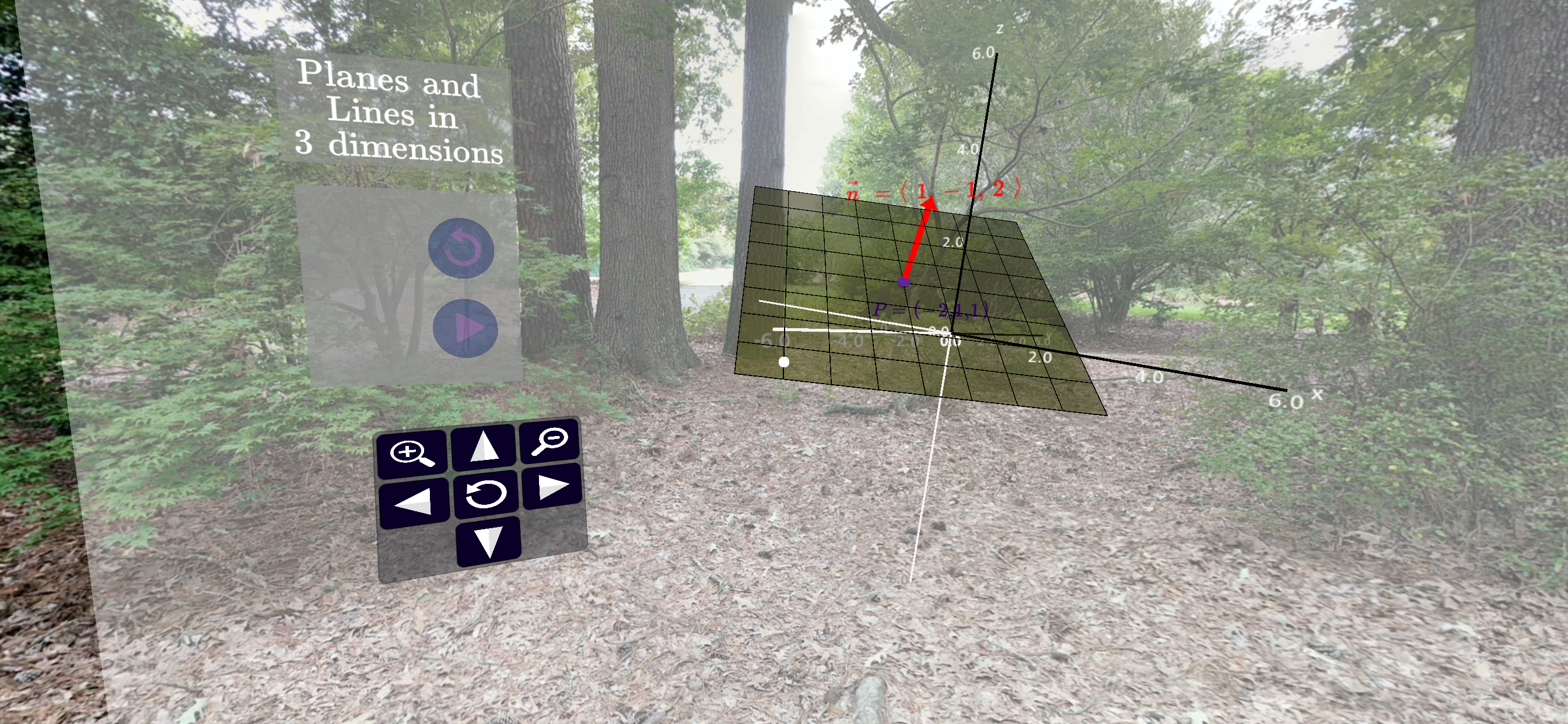
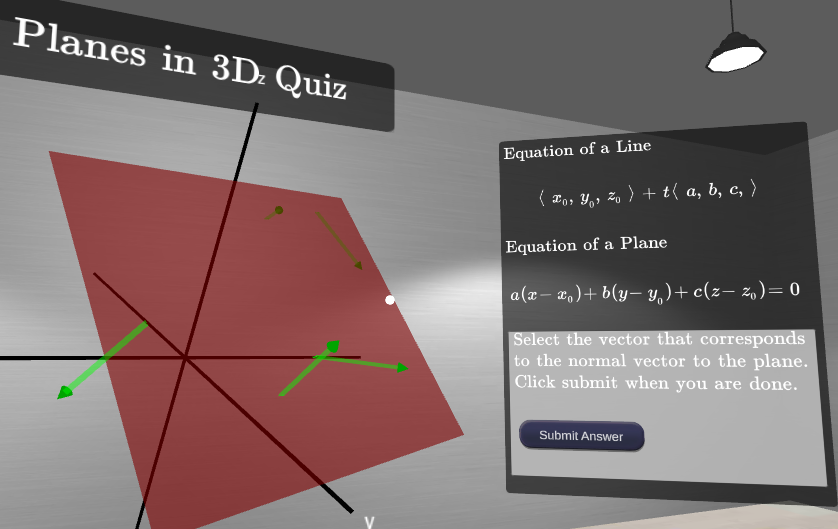
Consider the two equations for the planes:
\begin{align}
2x-y+3z & =4 \tag{3.4.3}\\
x-3y+z & =-3 \notag
\end{align}
Multiplying the second equation by \(-3\) we get
\begin{align*}
2x-y+3z & =4 \\
-3x+9y-3z & =9
\end{align*}
Adding these equations together we get an equation of two variables
\begin{equation*}
-x+8y = 13.
\end{equation*}
So we need to find \(x,y\) that satisfy the above. There are a lot of ways to accomplish this. You could pick your favorite number and plug it in for either \(x\) or \(y\text{.}\) Afterwards you could solve for the other variable. Let’s use this strategy and let \(y=2\text{.}\) The above equation reduces to
\begin{equation*}
-x+16 =13
\end{equation*}
and thus we get \(x=3\text{.}\) At this point we have \(x=3\) and \(y=2\text{.}\) To find the value for \(z\) we can use either of the plane equations in (3.4.3). We use the first one and substitute our values for \(x\) and \(y\) to achieve
\begin{equation*}
2(3)-2+3z = 4,
\end{equation*}
which gives us \(z=0\text{.}\) We have found the point \((3,2,0)\text{.}\) To verify the point \((3,2,0)\text{,}\) we substitue the values into both the plane equation found in equation (3.4.3). When we do this we get
\begin{align*}
2(3)-2+3(0) & =4 \\
-3(3)+9(-2)-3(0) & =9.
\end{align*}
Since both equation are satisfied, the point \((3,2,0)\) is on both planes and thus on the line of intersection of the planes.
(f)
Use the point and direction vector from the previous parts to find the equation of the line where the planes from equation (3.4.2) intersect. Use the tool below confirm your results. (Note: you must enter the times symbol "*". For example, "2t" will not work, but "2*t" will.)
Exercises 3.4.4 Exercises
For the following find the equation of the line where the planes intersect. Confirm your results using the tool above.
1.
\begin{align*}
3x-2y+z & =4 \\
-2x+5y-2z & =9
\end{align*}
2.
\begin{align*}
6x-7y+4z & =10 \\
-4x+6y+3z & =-8
\end{align*}
3.
\begin{align*}
2(x-3)-4(z+1) & =0 \\
4(x+2)-3(y-2)+5(z-3) & =17
\end{align*}
Subsection 3.4.5 Planes Quiz
This quiz generates a plane and the user is asked several questions about the line presented.
